Chapter 1: Introduction to Infinite Products

Have you ever encountered a mathematical expression that seems to stretch on indefinitely? In this section, we will explore how to simplify such infinite expressions using algebraic techniques.

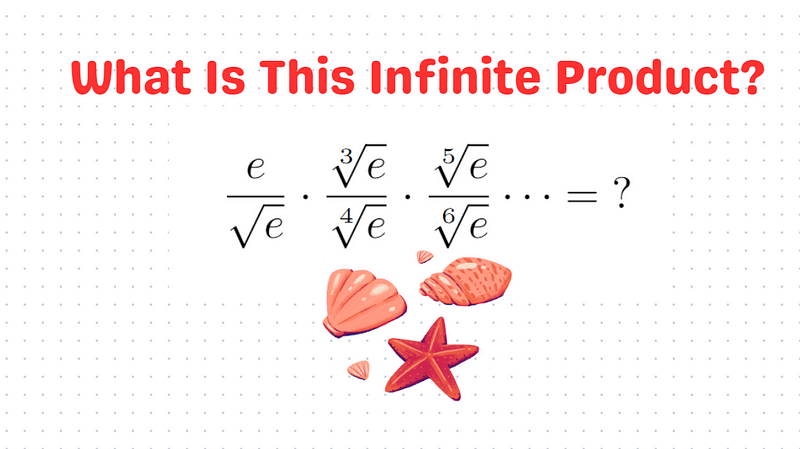
The concept of infinite products can be both fascinating and challenging. To effectively simplify an infinitely long expression, a solid understanding of the series expansion of natural logarithms is essential.

Before diving deeper, I encourage you to pause, grab some paper and a pen, and try to tackle this problem on your own. When you're ready, continue reading for the solution!

Section 1.1: The Solution Process

To solve the infinite product, we start by rewriting the expression in a manageable form, as demonstrated in the image below.

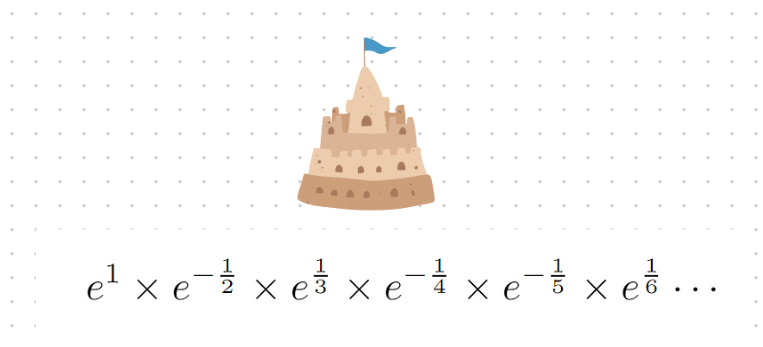
Next, we can combine the indices of the terms involved.

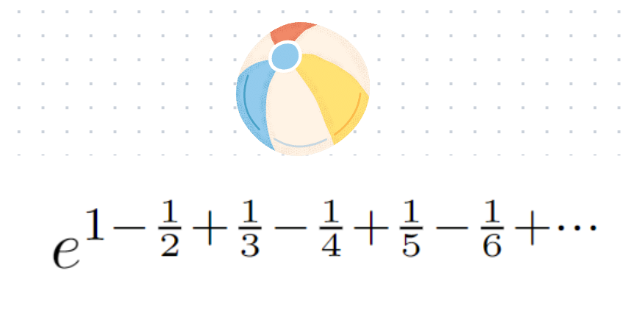
Utilizing the series expansion of the natural logarithm allows us to effectively approach this infinite product problem.

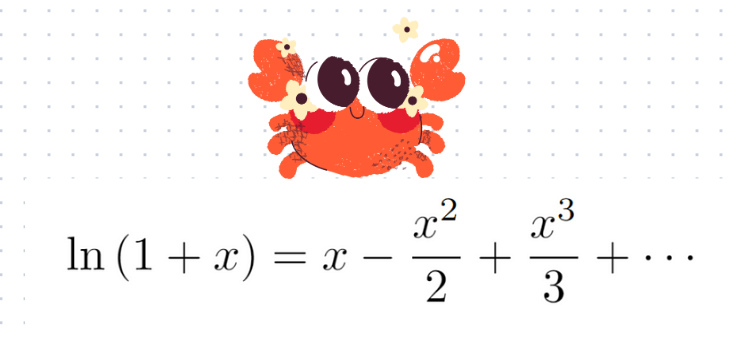
It's crucial to notice that we can replace x with 1 in the expansion we have derived.

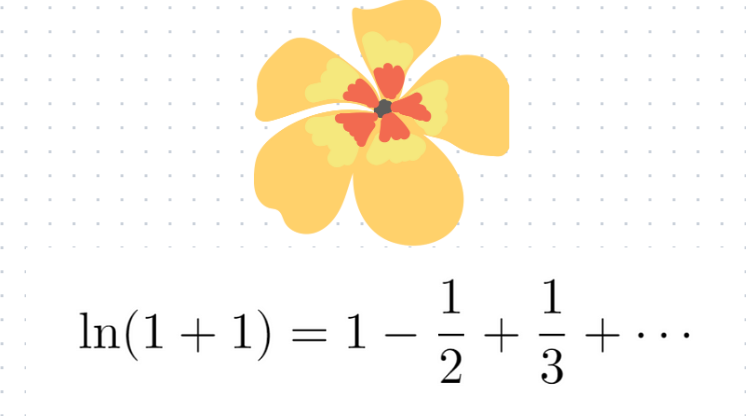
With this substitution, the initial query transforms into a more straightforward expression.

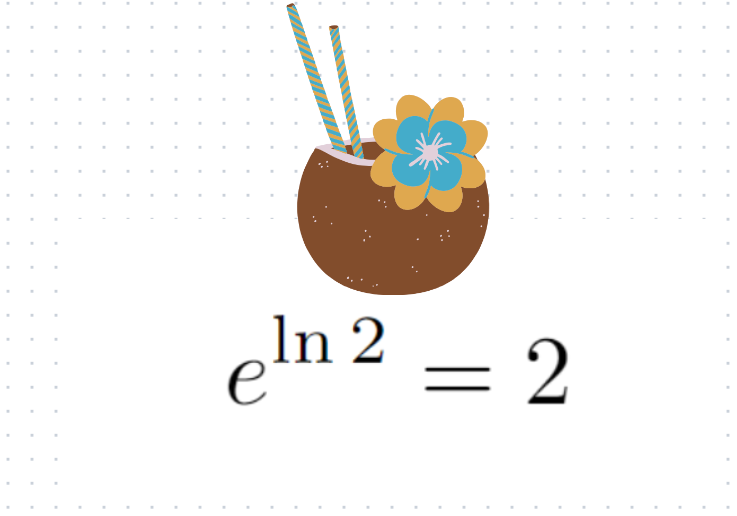
This process leads us to our final answer, which is a result of the simplification we performed.

Photo by Sean Oulashin on Unsplash

Isn't it amazing how we can simplify such complex concepts?

What was your approach to solving this problem? I would love to hear your thoughts in the comments below!

Chapter 2: Additional Resources

To further enhance your understanding of infinite products, I recommend checking out the following videos:

The first video titled "Infinite Series vs. Infinite Product" provides a comprehensive overview of the distinctions and similarities between these two mathematical concepts.

In the second video, "08a - Infinite Products," you'll find a deeper exploration of infinite products, offering practical examples and techniques to master them.

If you enjoyed this content and found it helpful, please consider sharing it with friends and fellow math enthusiasts.

Thank you for taking the time to read this article! Your support means a lot to me. If you would like to receive notifications for future publications, feel free to sign up.